Introduction: Why is Bending Radius Important?

In power engineering, excessive cable bending can lead to insulation damage, conductor breakage, and other hidden dangers. A nuclear power plant once experienced a short circuit due to insufficient cable bending radius, resulting in direct losses exceeding one million dollars. Correctly calculating the bending radius is a key technology for ensuring cable longevity and safe operation.

How to Calculate the Bending Radius of the Cable?

I. Core Concept Analysis

1. Definition of Bending Radius: The radius of the inner arc (R) when a cable is bent, directly related to the cable's outer diameter (D)

2. Minimum Bending Radius: The minimum allowable value that ensures the cable's structural integrity, typically a multiple of D

3. Destructive Bending Case Study: A wind farm using a 6×D bending radius led to armor layer deformation, reducing cable life by 40%

II. Key Influencing Factors

1. Cable Structure (coefficient comparison):

   • Single-core non-armored: 12×D

   • Multi-core armored: 15×D

   • Fiber composite cable: 20×D

2. Material Characteristics:

   • PVC insulation layers endure 30% fewer bending cycles than XLPE

   • Aluminum alloy conductors have 15% higher bending elasticity than copper conductors

3. Environmental Parameters:

   • Low temperature (-20°C) requires a 20% increase in bending radius

   • Dynamic bending conditions require 1.5 times larger radius than static conditions

III. International Standards and Calculation Formulas

1.IEC 60287 Standard: Rmin = K×D K value table:

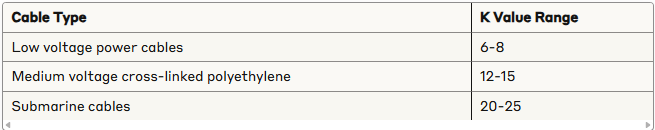
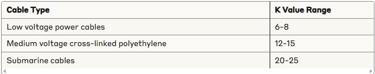
2.Dynamic Bending Formula: R = (E×I)/(M×σ)

E: Elastic modulus

I: Sectional moment of inertia

σ: Allowable stress

3.Engineering Quick Calculation Techniques:

Palm rule: Adult palm length ≈ 15×D (suitable for 10kV cables)

Conduit verification: Conduit diameter ≥ 10× cable diameter

IV. Practical Steps (Using a 10kV Armored Cable as an Example)

1. Measure the outer diameter: Use a π tape to obtain D=58mm

2. Check standards to determine K value: Choose 15 for armored multi-core cables

3. Calculate Rmin = 15×58 = 870mm

4. Installation control:

   • Use a guide wheel with 900mm radius

   • Set bending limit markers

   • Verify with a thermal imaging camera that temperature rise at bending points is < 3°C

V. Common Errors and Solutions

1. Error: Bending multiple cables together as a bundle Improvement: Adopt layered offset bending with spacing maintained at 2×D

2. Error: Ignoring slack cable coiling radius Solution: When using serpentine layouts, amplitude should be > 5×Rmin

3. Error: Using sharp angle bends Solution: Use three-section 135° bends in combination instead of 90° right angles

VI. Smart Detection Technology

1. Fiber-optic Sensing: Embed strain-sensing optical fibers for real-time bending deformation monitoring

2. 3D Laser Scanning: Build curved surface models with deviation detection accuracy of ±2mm

3. Machine Learning Prediction: Life prediction models based on historical data with accuracy > 85%

Conclusion

Mastering bending radius calculations requires combining theory with practice. A cross-sea bridge project achieved less than 1% bending radius error over 3,000 meters of continuous cable installation by using precise calculations, setting a new industry benchmark. Engineers are recommended to carry quick calculation cards with Rmin values of commonly used cables in tabular form to improve on-site work efficiency.